IBM® SPSS® Amos™ 28

In this example, BootBS requests a Bollen-Stine bootstrap analysis, and Bootstrap 2000 requests 2000 bootstrap samples.

The Bollen-Stine procedure provides a test of the hypothesis that the model is correct. This is the same null hypothesis that is tested by the conventional chi-square test of fit in maximum likelihood, generalized least squares and asymptotically distribution-free estimation. The objective of the procedure is to ascertain the probability that the discrepancy function would be as large as it actually turned out to be in the current sample, under the hypothesis that your model is correct.

In the Bollen-Stine approach, a transformation of the sample data is carried out so as to make your model fit the transformed data exactly. Bootstrap samples are drawn from the transformed sample data. The distribution of the discrepancy function across bootstrap samples is then taken as an estimate of its distribution under the hypothesis that the model is correct.

Using maximum likelihood estimation (Amos's default), the likelihood ratio chi-square statistic is 7.853 with 8 degrees of freedom (p = .448). The following output indicates that 46.2% of the 2000 bootstrap samples had a likelihood ratio chi-square statistic greater than 7.853.

    Testing the null hypothesis that the specified model is correct:
              Bollen-Stine bootstrapped p = 0.462

Thus, the departure of the data from the model is significant at the .462 level. In other words, the data do not depart significantly from the model at any conventional significance level. The distribution of 2000 likelihood ratio chi-square statistics obtained from the 2000 bootstrap samples is as follows.

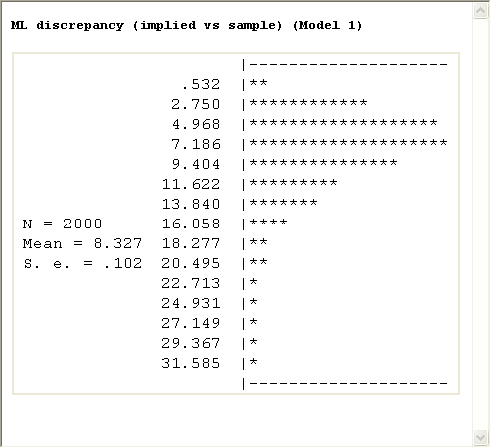
This distribution resembles the chi-square distribution with eight degrees of freedom insofar as it is positively skewed and has a mean of about eight (actually 8.327). Unfortunately, Amos does not provide the information needed to do a more detailed comparison with the chi-square distribution.